Study Notes

Two blocks $A$ and $B$ of mass $m_{A}$ and $m_{B}$ respectively are kept in contact on a frictionless table. The experimenter pushes the block $A$ from behind so that the blocks accelerate. If the block $A$ exerts a force $F$ on the block $B$, what is the force exerted by the experimenter on $A$ ?

(A) $F\left( {\frac{{{m_B}}}{{{m_A}}}} \right)$
(B) $F\left( {\frac{{{m_A}}}{{{m_B}}}} \right)$
(C) $F\left( 1+{\frac{{{m_B}}}{{{m_A}}}} \right)$
(D) $F\left(1+ {\frac{{{m_A}}}{{{m_B}}}} \right)$

The correct answer is (D) $F\left(1+ {\frac{{{m_A}}}{{{m_B}}}} \right)$.

Solution:

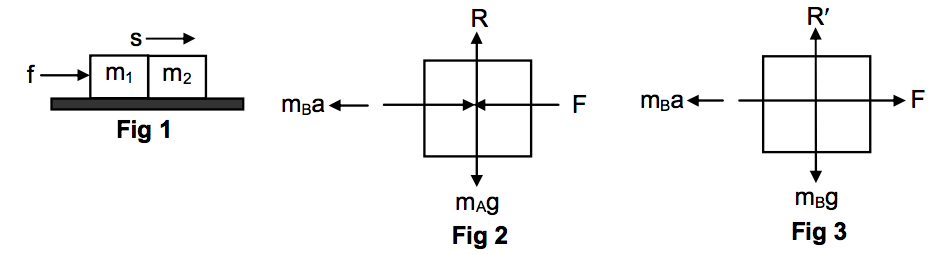
Let, $F \rightarrow$ contact force between $m_{A} \& m_{B}$

And, $f \rightarrow$ force exerted by experimenter.

$F+m_{A} a-f=0$
$\Rightarrow \mathrm{F}=\mathrm{f}-\mathrm{m}_{\mathrm{A}} \mathrm{a} \quad \quad \ldots (i)$

$\mathrm{m}_{\mathrm{B}} \mathrm{a}-\mathrm{f}=0$
$\Rightarrow \mathrm{F}=\mathrm{m}_{\mathrm{B}} \mathrm{a} \quad \quad \ldots (ii)$

From eqn $(i)$ and eqn $(ii)$

$\Rightarrow f-m_{A} a=m_{B} a \Rightarrow f=m_{B} a+m_{A} a \Rightarrow f=a\left(m_{A}+m_{B}\right)$

$\Rightarrow f=\frac{F}{m_{B}}\left(m_{B}+m_{A}\right)=F\left(1+\frac{m_{A}}{m_{B}}\right)\left[\text { because } a=F / m_{B}\right]$

$\therefore$ The force exerted by the experimenter is $\mathrm{F}\left(1+\frac{\mathrm{m}_{\mathrm{A}}}{\mathrm{m}_{\mathrm{B}}}\right)$

JEE Question Bank

For best collection of JEE Main, JEE Advanced, Class 11 – 12 boards question visit ThinkMerit question bank.

JEE Mock Test

JEE Main, JEE Advanced unlimited mock test. Guaranteed help. Try yourself and witness the difference.